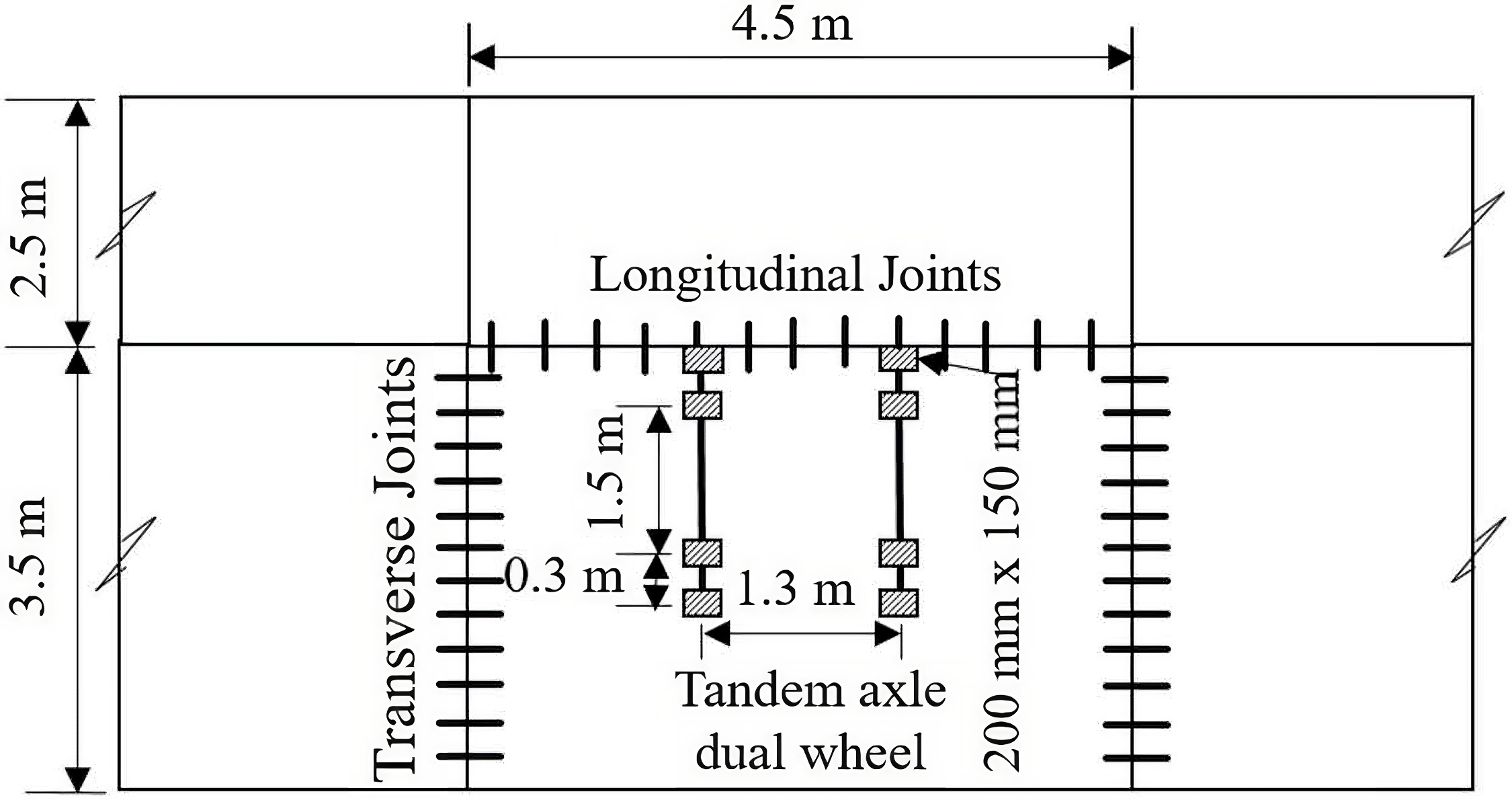
Cracking is considered one of the main reasons for the degradation of Pavement Quality Concrete (PQC) slab. The joints are provided along the PQC slab to restrict the formation of cracking in the PQC slab. In this study, a PQC slab with longitudinal, and transverse joints was modeled using a three-dimensional finite element-based software EverFE2.26. A multi-slab PQC (tandem axle dual wheel conditions) was modeled to take into account the practical conditions of the real pavement. An attempt has been made to consider the effect of varying surface temperatures on maximum tensile stress along with the other factors affecting the performance of the concrete pavement. The dowel group action was studied along with the dowel shear and vertical deflection with and without concrete shoulder. The critical stress was analyzed for a three-slab system with a tied concrete shoulder. It was observed that varying surface temperature (ST) does not affect the maximum tensile stress (MTS) for a multi-slab system. Single slab systems without any dowel bars, tie bars, and reinforcement experienced an increase in MTS with an increase in ST. The temperature variation leads to the contraction and expansion of concrete that potentially will lead to cracks. Therefore, the effect of ST on MTS cannot be neglected in such cases. However, slabs connected by the dowel and tie bars did not experience a rise in MTS with the rise in ST. The dowel and tie bars provided in the joints not only reduce the cracking of slabs but also minimized the tensile stress in the concrete pavement. A regression model was developed to estimate the maximum tensile stress for varying temperature differentials, slabs, axles, and dowel parameters. The R2 value of the regression model was obtained as 0.863. The validation of the regression model showed that the differences between predicted and actual stresses obtained from EverFE2.26 were less than 10%.
This model may be used directly to estimate the maximum tensile stress in the concrete pavement with varying parameters. However, further refinement of the model may be carried out for multi-slab systems with or without reinforcement and tie bars.